The evolution of trust

A wonderful serious game developed by Nicky Case on game theory and trust emergence phenomena: https://ncase.me/trust/

Complete the sequence

1
11
21
1211
111221
?

Four men in hats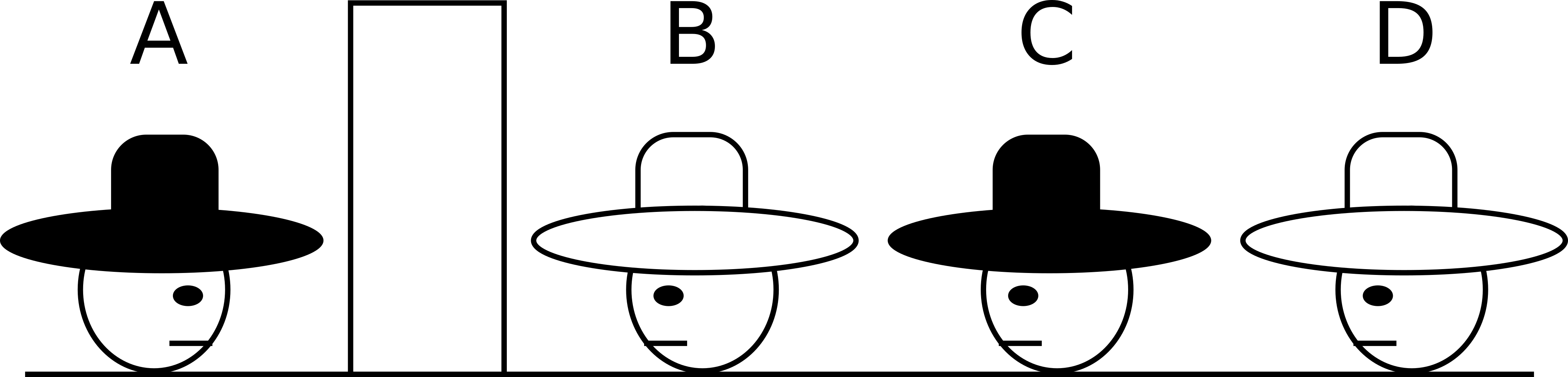

Four men are buried in line up to their necks in the ground. They cannot move and can only look forward. Between A and B is a brick wall which cannot be seen through. They all know that they are wearing four hats --two black and two white-- but they do not know what color is their own hat. In order to escape, one of them must call out to the executioner the color of their hat. If they get it right, they are free. They are not allowed to talk to each other and have 10 minutes to figure it out.
After one minute, one of them calls out.
Which one of them calls out and how did he understand?

The sick monks

Once upon a time there was a monastery built atop a mountain in which one hundred monks live isolated from the rest of the world. The rule of the monastery is quite strict: monks cannot communicate with each other, whether it is through writing, talking or any body language. No communication at all. They spend the day working and praying alone but dine together every evening.
One day, the master of the monastery decides to exceptionally break the rule to inform his fellow monks of one important fact.
There is an illness among us. It is fortunately not contagious nor particularly dangerous, but I would like every sick person to remain in bed for an entire day. The illness has only one recognizable symptom: it causes an easily visible red dot to appear on one's forehead.
The master did not point out the sick monks and there are no mirrors in the monastery.
Seven day pass without a single monk missing. After the seventh day (on the eighth day), all sick monks leave the dinner table to go rest in bed just as they were instructed.
How many monks were ill and how did they find out?

The gate keepers

After a long journey, you arrive at last in front of the gate leading to the Atlantis treasure. But, to your surprise, there is not only one but two gates and both are protected by giant stone soldiers. Above the two gates, you can read the following.
One door to Death.
One door to Wealth.
One soldier of Lie.
One soldier of Truth.
On one question you rely.
For yes or no will get you through.
Which question will you ask to which guard to know which gate leads to the treasure?

The blue coins

At the time, joking about the king sounded like a very good idea. At the time. Now you are trapped in a room of the royal castle, in total darkness. You are free to go at any time though. However you will be able to do so with your head still on your neck at one condition: the number of blue-top coins outside and inside the room must be equal when you leave. The room contains indeed a certain number of coins which have a blue side and a red one. When you entered the room, you were told that there were exactly 5 coins with the blue side on top. All the coins are initially in the room, no coin outside of it. You can do and take whatever you want in the room. However, you are in total darkness and the coins cannot be destroyed.
What can you do to succeed this challenge?